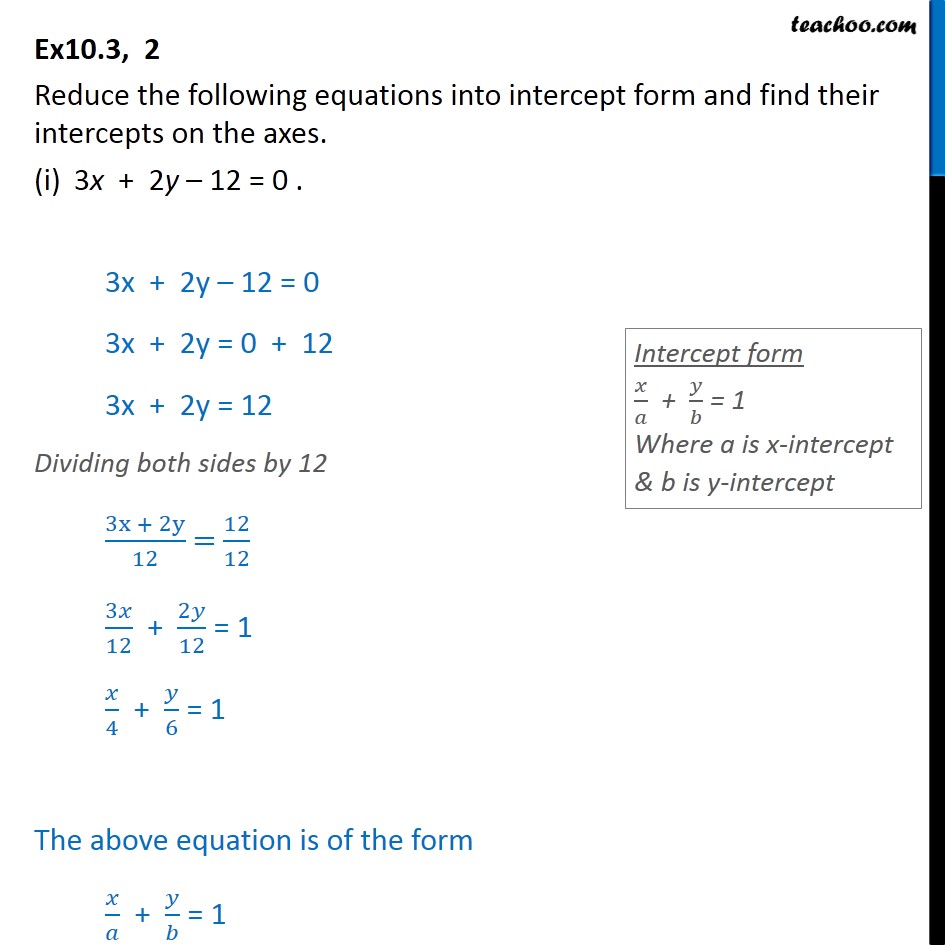
3x 2y 6 In Slope Intercept Form
Slope-intercept Form of a Line
Slope-intercept form is one of the most important forms of linear equations and is used to graph lines in two-dimensions. The equation of a line in slope-intercept form is expressed as y = mx + b, where m is the slope and b is the y-intercept. In this article, we'll look at how to solve the equation 3x + 2y = 6 in slope-intercept form.
Solving 3x + 2y = 6 in Slope-intercept Form
The first step in solving for the equation for 3x + 2y = 6 is to move all the terms to one side and make the other side equal to zero. To do this, we subtract 3x from each side and subtract 2y from each side, giving us 2y = -3x + 6.
Next, we'll divide both sides of the equation by 2 to get y = -(3/2)x + 3. This tells us that the slope is -(3/2) and the y-intercept is 3.
Using the Slope-intercept Form
Now that we have the equation for 3x + 2y = 6 in slope-intercept form, we can use it to graph the line. To graph the line, we'll start with the y-intercept and plot it on a graph. In this case, the y-intercept is 3, so we'll plot the point (0, 3).
Next, we'll use the slope, -(3/2) to find another point on the line. To do this, we'll use the point-slope formula, which is y - y1 = m(x - x1). We'll use the point (0, 3) as our starting point, so x1 = 0, y1 = 3. We'll also use the slope, -(3/2). When plugged into the formula, we get y - 3 = -(3/2)(x - 0), or y - 3 = -(3/2)x. We can solve this equation for y to get y = -(3/2)x + 3.
Conclusion
In conclusion, the equation 3x + 2y = 6 can be rewritten in slope-intercept form, which is y = -(3/2)x + 3. This equation can then be used to graph the line on a two-dimensional graph. With slope-intercept form, graphing lines in two-dimensions is easier than ever.
Graph 3x + 2y = 6 - YouTube
Use the slope-intercept form to graph the equation 3x+2y=6 - Brainly.com

Ex 10.3, 2 - Reduce the equation 3x + 2y - 12 = 0 into intercept form